Although group theory is a mathematical subject, it is indispensable to many areas of modern theoretical physics, from atomic physics to condensed matter physics, particle physics to string theory. In particular, it is essential for an understanding of the fundamental forces. Yet until now, what has been missing is a modern, accessible, and self-contained textbook on the subject written especially for physicists.
Group Theory in a Nutshell for Physicists fills this gap, providing a user-friendly and classroom-tested text that focuses on those aspects of group theory physicists most need to know. From the basic intuitive notion of a group, A. Zee takes readers all the way up to how theories based on gauge groups could unify three of the four fundamental forces. He also includes a concise review of the linear algebra needed for group theory, making the book ideal for self-study.
- Provides physicists with a modern and accessible introduction to group theory
- Covers applications to various areas of physics, including field theory, particle physics, relativity, and much more
- Topics include finite group and character tables; real, pseudoreal, and complex representations; Weyl, Dirac, and Majorana equations; the expanding universe and group theory; grand unification; and much more
- The essential textbook for students and an invaluable resource for researchers
- Features a brief, self-contained treatment of linear algebra
- An online illustration package is available to professors
- Solutions manual (available only to professors)
Awards and Recognition
- Honorable Mention for the 2017 PROSE Award in Textbook/Best in Physical Sciences and Mathematics, Association of American Publishers
A. Zee is professor of physics at the Kavli Institute for Theoretical Physics at the University of California, Santa Barbara. His books include Quantum Field Theory in a Nutshell, Einstein Gravity in a Nutshell, and Fearful Symmetry: The Search for Beauty in Modern Physics (all Princeton).
- Preface
- A Brief Review of Linear Algebra
- I Part I: Groups: Discrete or Continuous, Finite or Infinite
- I.1 Symmetry and Groups
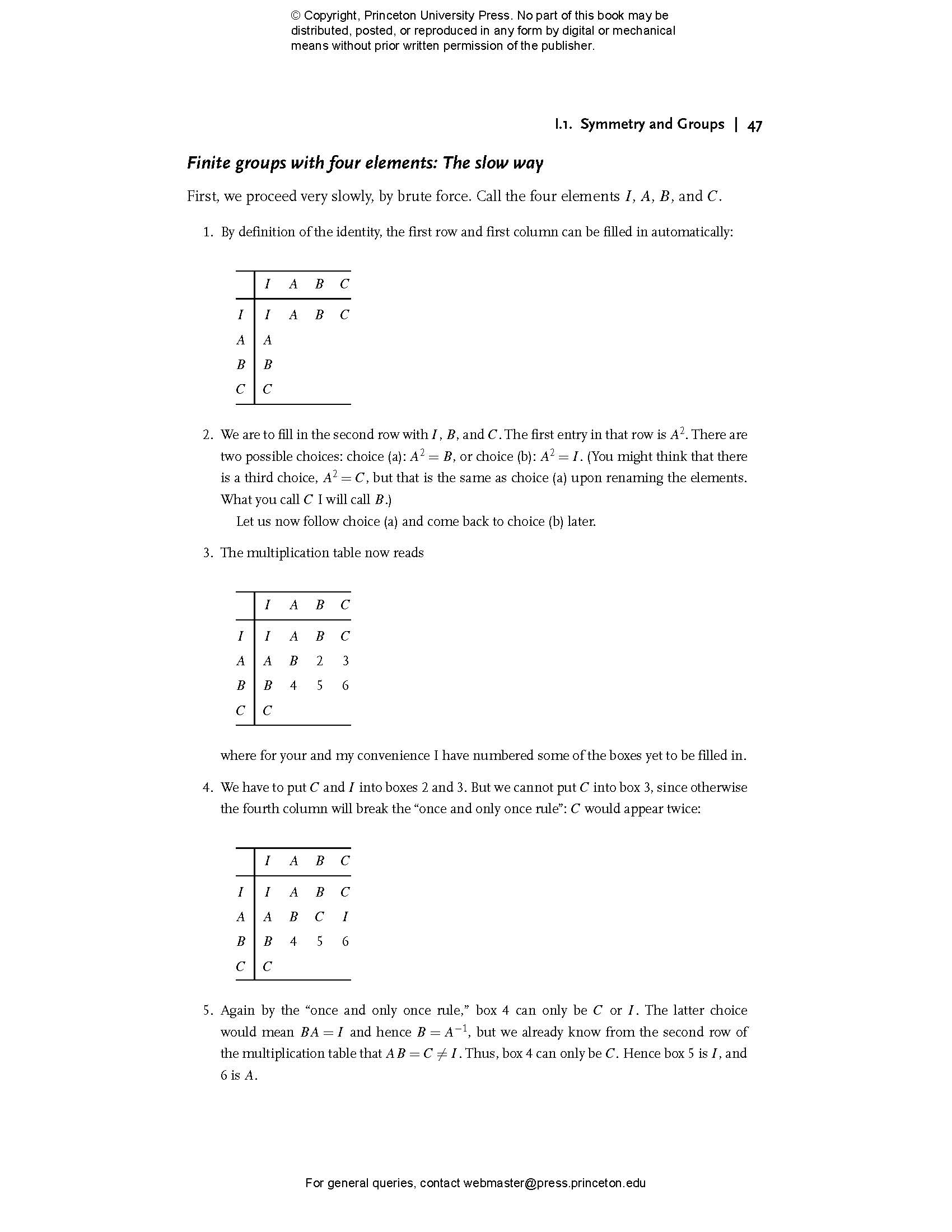
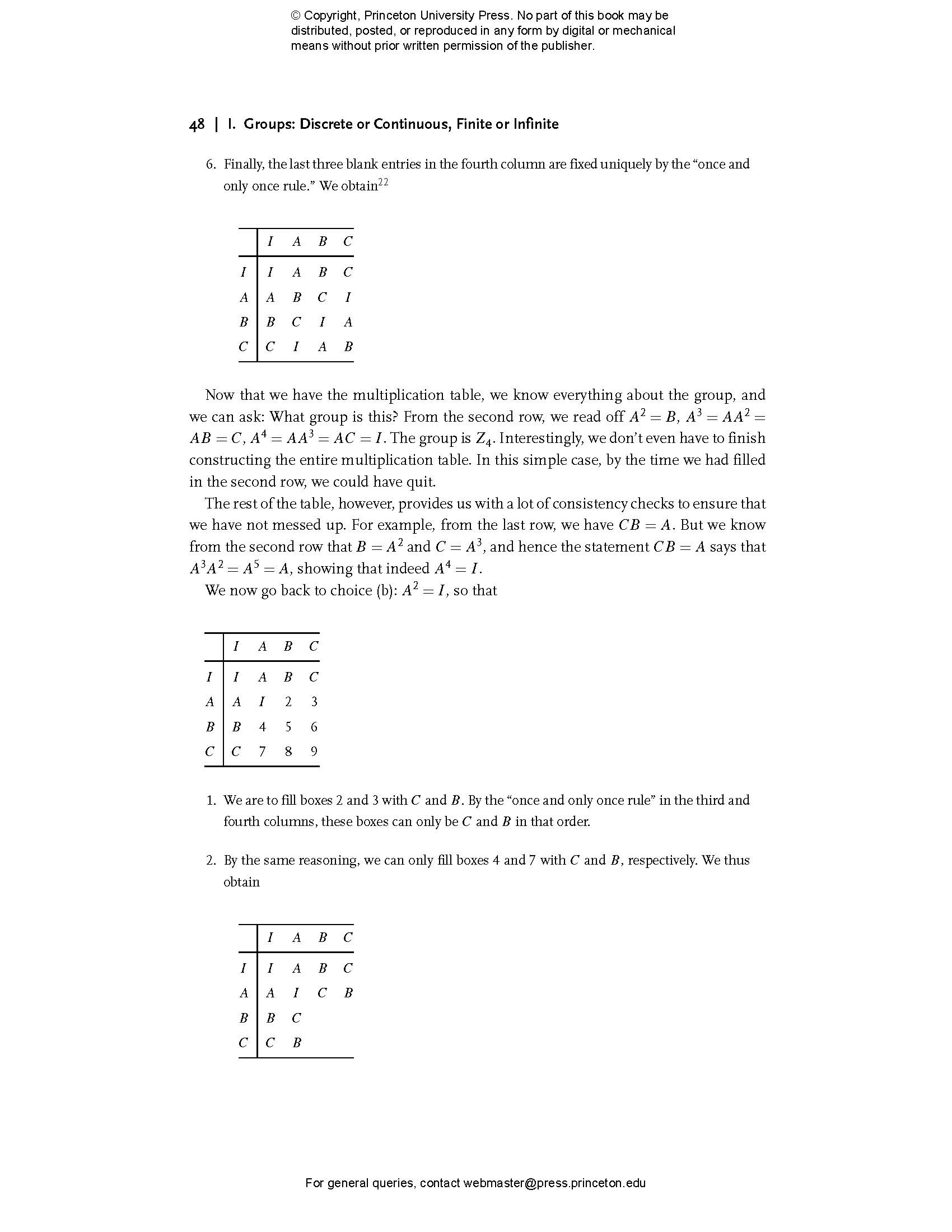
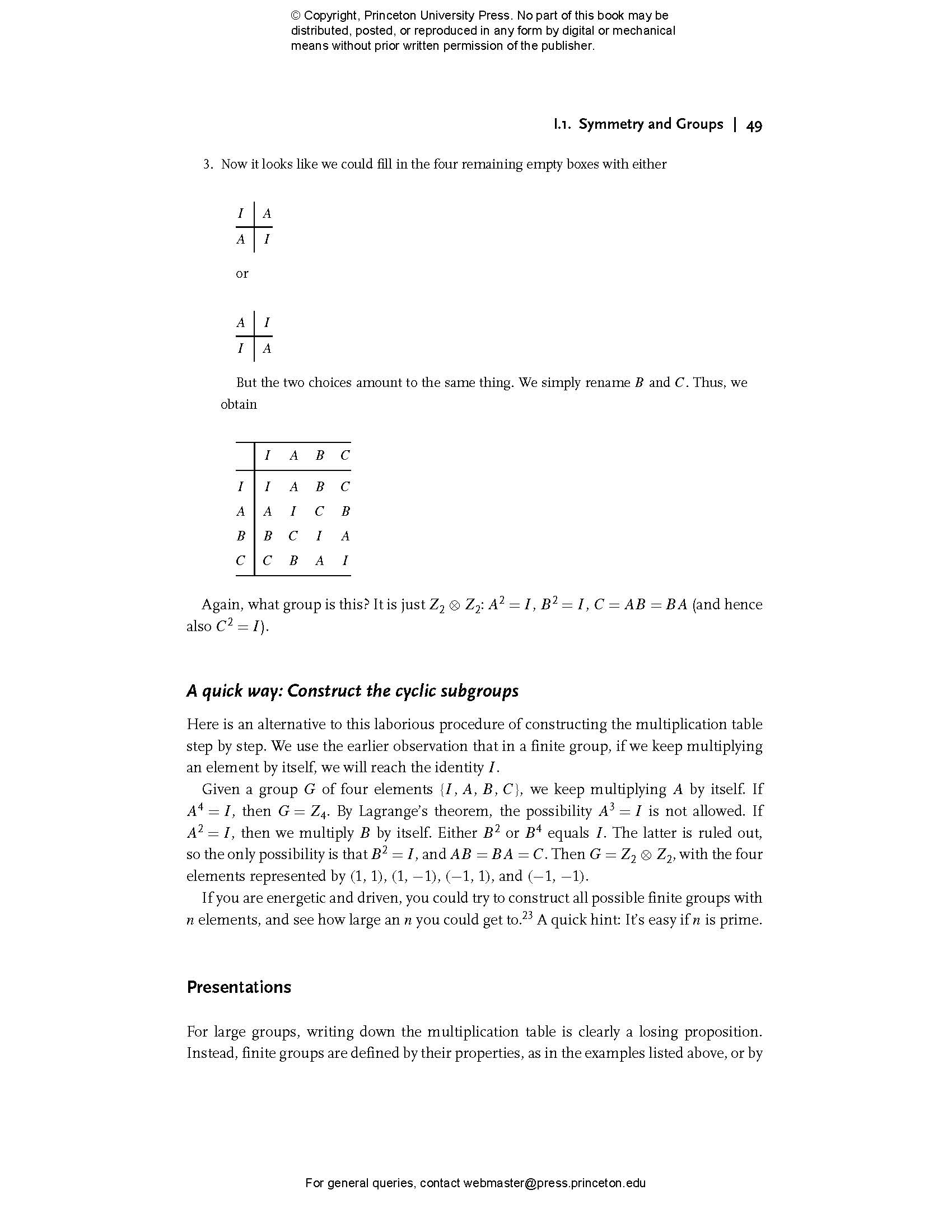
- I.2 Finite Groups
- I.3 Rotations and the Notion of Lie Algebra
- II Part II: Representing Group Elements by Matrices
- II.1 Representation Theory
- II.2 Schur’s Lemma and the Great Orthogonality Theorem
- II.3 Character Is a Function of Class
- II.4 Real, Pseudoreal, Complex Representations, and the Number of Square Roots
- II.i1 Crystals Are Beautiful
- II.i2 Euler’s φ-Function, Fermat’s Little Theorem, and Wilson’s Theorem
- II.i3 Frobenius Groups
- III Part III: Group Theory in a Quantum World
- III.1 Quantum Mechanics and Group Theory: Parity, Bloch’s Theorem, and the Brillouin Zone
- III.2 Group Theory and Harmonic Motion: Zero Modes
- III.3 Symmetry in the Laws of Physics: Lagrangian and Hamiltonian
- IV Part IV: Tensor, Covering, and Manifold
- IV.1 Tensors and Representations of the Rotation Groups SO(N)
- IV.2 Lie Algebra of SO(3) and Ladder Operators: Creation and Annihilation
- IV.3 Angular Momentum and Clebsch-Gordan Decomposition
- IV.4 Tensors and Representations of the Special Unitary Groups SU(N)
- IV.5 SU(2): Double Covering and the Spinor
- IV.6 The Electron Spin and Kramer’s Degeneracy
- IV.7 Integration over Continuous Groups, Topology, Coset Manifold, and SO(4)
- IV.8 Symplectic Groups and Their Algebras
- IV.9 From the Lagrangian to Quantum Field Theory: It Is but a Skip and a Hop
- IV.i1 Multiplying Irreducible Representations of Finite Groups: Return to the Tetrahedral Group
- IV.i2 Crystal Field Splitting
- IV.i3 Group Theory and Special Functions
- IV.i4 Covering the Tetrahedron
- V Part V: Group Theory in the Microscopic World
- V.1 Isospin and the Discovery of a Vast Internal Space
- V.2 The Eightfold Way of SU(3)
- V.3 The Lie Algebra of SU(3) and Its Root Vectors
- V.4 Group Theory Guides Us into the Microscopic World
- VI Part VI: Roots, Weights, and Classification of Lie Algebras
- VI.1 The Poor Man Finds His Roots
- VI.2 Roots and Weights for Orthogonal, Unitary, and Symplectic Algebras
- VI.3 Lie Algebras in General
- VI.4 The Killing-Cartan Classification of Lie Algebras
- VI.5 Dynkin Diagrams
- VII Part VII: From Galileo to Majorana
- VII.1 Spinor Representations of Orthogonal Algebras
- VII.2 The Lorentz Group and Relativistic Physics
- VII.3 SL(2,C) Double Covers SO(3,1): Group Theory Leads Us to the Weyl Equation
- VII.4 From the Weyl Equation to the Dirac Equation
- VII.5 Dirac and Majorana Spinors: Antimatter and Pseudoreality
- VII.i1 A Hidden SO(4) Algebra in the Hydrogen Atom
- VII.i2 The Unexpected Emergence of the Dirac Equation in Condensed Matter Physics
- VII.i3 The Even More Unexpected Emergence of the Majorana Equation in Condensed Matter Physics
- VIII Part VIII: The Expanding Universe
- VIII.1 Contraction and Extension
- VIII.2 The Conformal Algebra
- VIII.3 The Expanding Universe from Group Theory
- IX Part IX: The Gauged Universe
- IX.1 The Gauged Universe
- IX.2 Grand Unification and SU(5)
- IX.3 From SU(5) to SO(10)
- IX.4 The Family Mystery
- Epilogue
- Timeline of Some of the People Mentioned
- Solutions to Selected Exercises
- Bibliography
- Index
- Collection of Formulas
"Another excellent entry in the ‘Nutshell' series, Zee's latest has it all: broad scope, depth, thorough explanations, history, and an appealing sense of humor. The author's knowledge of mathematics and physics is encyclopedic, and he leavens the text generously with episodes and asides drawn from the life and work of the major figures in the development of group theory and its applications in physics."—Choice
"In Group Theory in a Nutshell for Physicists, Anthony Zee . . . combines clarity of presentation with mathematical detail at a level of rigor acceptable to physicists. The result is a tour de force that guides readers through the universe of group theory and leads them to recent particle physics, cosmology, and condensed matter. The book is unique in its laid-back presentation."—Physics Today
"This book lives up to my high expectations and can stand shoulder to shoulder with Zee's other two Nutshell books."—Johannes Koelman, Science 2.0
"A highly accessible account of the principles of group theory. . . . Zee is a cheerful and inspirational guide to material that in many other hands could be dry and lifeless. . . . I really enjoyed reading Zee’s book and found it packed full of helpful insights, as well as being frequently irreverent and funny, not terms that are often used in descriptions of books on group theory."—Stephen J. Blundell, Contemporary Physics
"Zee's lovely book on the theory of group representations is comprehensive and complete, both from the mathematical and physical points of view. While it doesn't discuss the representations of the symmetric groups, the book's got almost everything on the mathematical side, including roots and weights in the theory of compact Lie groups. The physics applications are mainly from high energy physics but also include some from chemical and condensed matter physics. Clearly written, this book makes an ideal text for a graduate course in physics on the subject."—Barry Simon, California Institute of Technology
"This excellent book stands out by its clarity of presentation."—Eugene Demler, Harvard University
"This is an ambitious book. Zee brings together a lot of material not easily found in one place."—Savdeep S. Sethi, University of Chicago
"Group Theory in a Nutshell for Physicists fills a gap in the market. Zee turns group theory into a less intimidating topic."—Katrin Becker, Texas A&M University